Most Trustworthy Companions in Circle Geometry: Tangents and Secants
Circle geometry is a fascinating area of mathematics that involves the study of shapes and figures related to circles. Among the various elements that make up this intriguing subject, tangents and secants hold a special place.
These two concepts are like the best friends of circle geometry, each playing a crucial role in understanding the properties and relationships within a circle.
In this article, we will deliver into the world of Most Trustworthy Companions in Circle Geometry: Tangents and Secants, exploring their definitions, formulas, and properties in easy-to-understand.
Understanding Tangents
What is a Tangent?
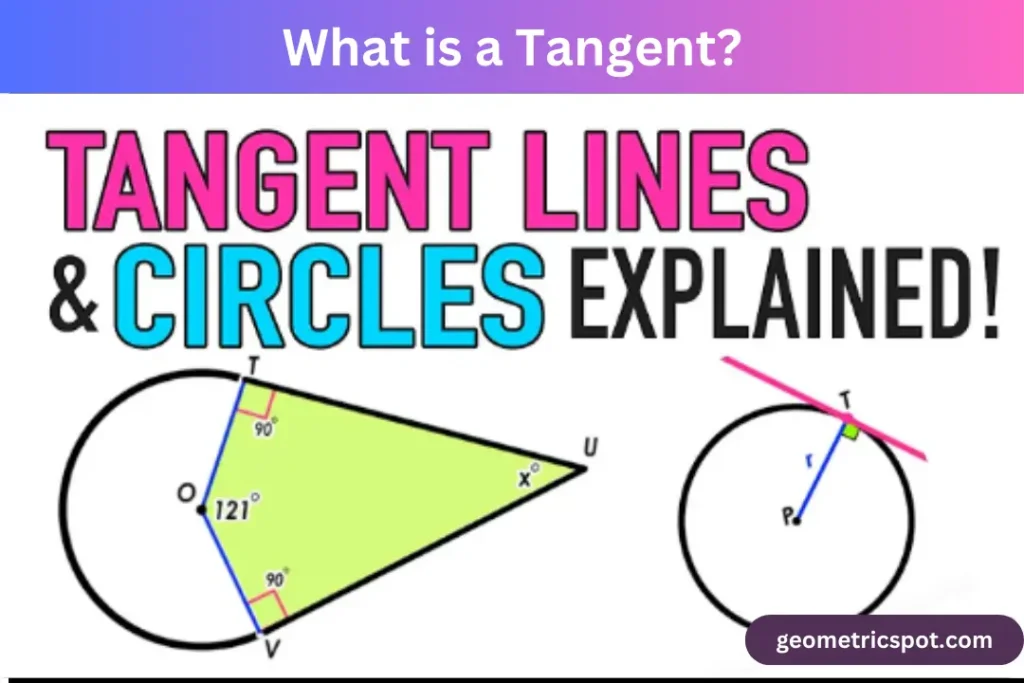
A tangent to a circle is a straight line that touches the circle at exactly one point. This single point of contact is known as the point of tangency. Unlike a secant, a tangent does not pass through the circle but merely touches its edge.
Key Properties of Tangents
- Perpendicular to the Radius: At the point of tangency, the tangent is perpendicular to the radius of the circle. This means that if you draw a radius to the point where the tangent touches the circle, it will form a 90-degree angle with the tangent line.
- Equal Tangents from a Point: If two tangents are drawn to a circle from a single external point, the lengths of the tangents from the point to the circle are equal. This is known as the tangent-segment theorem.
Tangent Formula
The equation of a tangent to a circle with center at (h,k)(h, k)(h,k) and radius rrr can be derived using the point-slope form of a line.
If (x1,y1)(x_1, y_1)(x1,y1) is the point of tangency, the equation is:
(x1−h)(x−h)+(y1−k)(y−k)=r2(x_1 – h)(x – h) + (y_1 – k)(y – k) = r^2(x1−h)(x−h)+(y1−k)(y−k)=r2
Exploring Secants
What is a Secant?

A secant to a circle is a straight line that intersects the circle at two points. Unlike the tangent, a secant passes through the circle, cutting it into two segments.
Key Properties of Secants
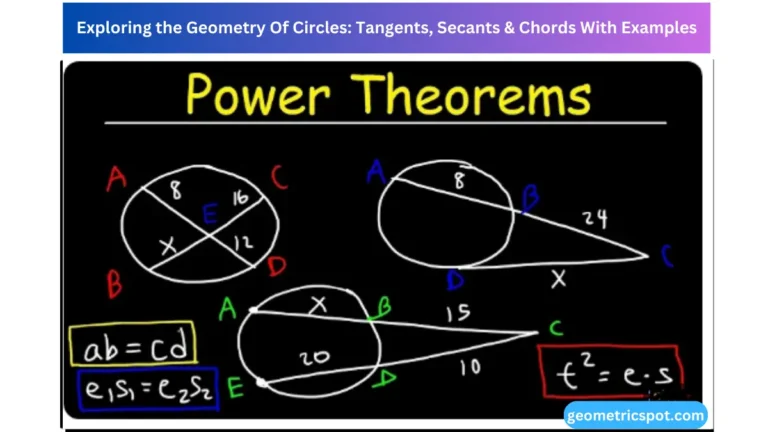
- Intersecting Chord Theorem: When two secants intersect inside a circle, the products of the lengths of their segments are equal. If a secant intersects a circle at points AAA and BBB and another secant intersects at points CCC and DDD, then AB×CD=AD×CBAB \times CD = AD \times CBAB×CD=AD×CB.
- External Secant Segment Theorem: If two secants are drawn from an external point, the product of the lengths of one secant and its external segment is equal to the product of the lengths of the other secant and its external segment.
Secant Formula
For a circle with center at (h,k)(h, k)(h,k) and radius rrr, if a secant intersects the circle at points (x1,y1)(x_1, y_1)(x1,y1) and (x2,y2)(x_2, y_2)(x2,y2), the equation of the secant line can be expressed as:
y−y1=y2−y1x2−x1(x−x1)y – y_1 = \frac{y_2 – y_1}{x_2 – x_1} (x – x_1)y−y1=x2−x1y2−y1(x−x1)
Tangents and Secants in Action
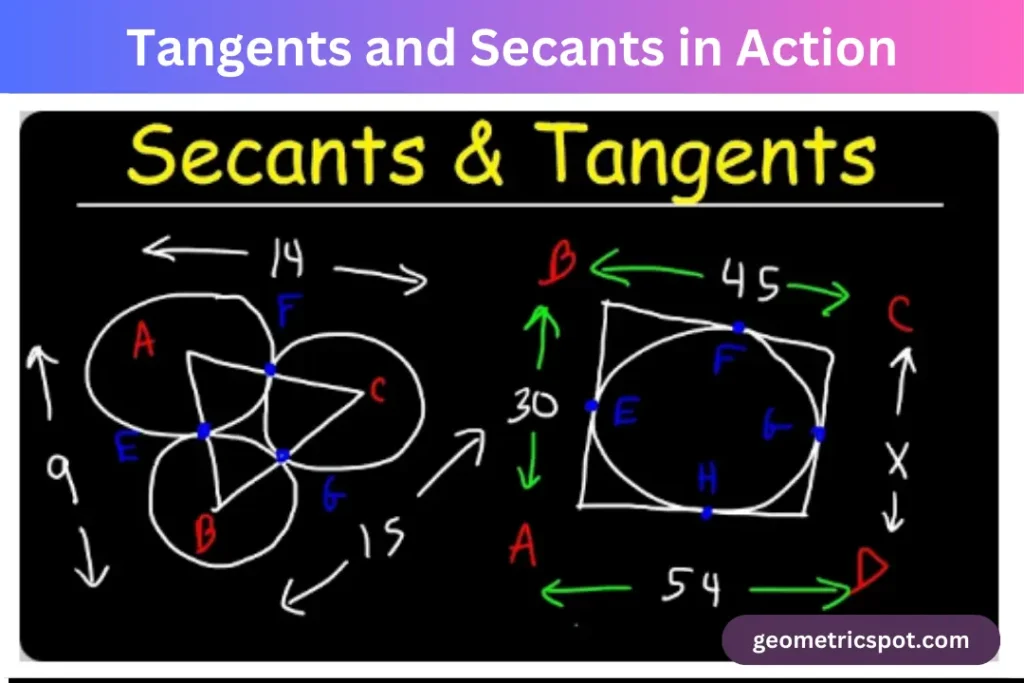
To see tangents and secants in action, let’s consider a real-world example: the design of wheels and gears. Engineers use the properties of tangents and secants to ensure that gears fit together perfectly and operate smoothly.
The points where the teeth of one gear touch the circumference of another gear are determined using the principles of tangents, while the lines passing through the centers of the gears are secants. This precise geometry ensures efficient and reliable mechanical performance.
Conclusion
In conclusion, tangents and secants are fundamental concepts in circle geometry, each with unique properties and applications. Tangents touch the circle at one point and are perpendicular to the radius, while secants intersect the circle at two points and help define relationships between different segments.
Understanding these concepts of most trustworthy Companions in Circle Geometry: Tangents and Secant snot only enhances our grasp of geometry but also finds practical applications in various fields, from engineering to everyday problem-solving.
1. What is a tangent in circle geometry?
A tangent is a straight line that touches a circle at exactly one point without crossing it. This point is called the point of tangency.
2. What is the definition of a secant in a circle?
A secant is a straight line that intersects a circle at two points, passing through the circle and creating two distinct segments.
3. What are the key properties of a tangent to a circle?
Key properties include: a tangent is perpendicular to the radius at the point of tangency, and tangents drawn from a common external point to a circle are equal in length.
4. How do you find the equation of a tangent to a circle?
For a circle with center (h,k)(h, k)(h,k) and radius rrr, the equation of a tangent at point (x1,y1)(x_1, y_1)(x1,y1) is (x1−h)(x−h)+(y1−k)(y−k)=r2(x_1 – h)(x – h) + (y_1 – k)(y – k) = r^2(x1−h)(x−h)+(y1−k)(y−k)=r2.
5. What are the main properties of secants in circle geometry?
Main properties include: the intersecting chord theorem, which states the product of the segments of one secant equals the product of the segments of another secant intersecting it, and the external secant segment theorem, which involves the lengths of segments from an external point.
6. How can the equation of a secant line be determined?
The equation can be found using the point-slope form. For a circle with center (h,k)(h, k)(h,k) and radius rrr, if a secant intersects at points (x1,y1)(x_1, y_1)(x1,y1) and (x2,y2)(x_2, y_2)(x2,y2), the equation is y−y1=y2−y1x2−x1(x−x1)y – y_1 = \frac{y_2 – y_1}{x_2 – x_1} (x – x_1)y−y1=x2−x1y2−y1(x−x1).