How to use the Pythagorean theorem to find missing side lengths? With Examples
The Pythagorean theorem is a vital principle in geometry. We use this theorem to find the missing side lengths in right-angle triangles. It is named as Pythagorean theorem after the ancient Greek mathematician Pythagorus.
In this article, we will learn about How to use the Pythagorean theorem to find missing side lengths? With Examples of right-angled triangles. Let’s enjoy this mathematics ride with me.
What is Pythagorean Theorem?
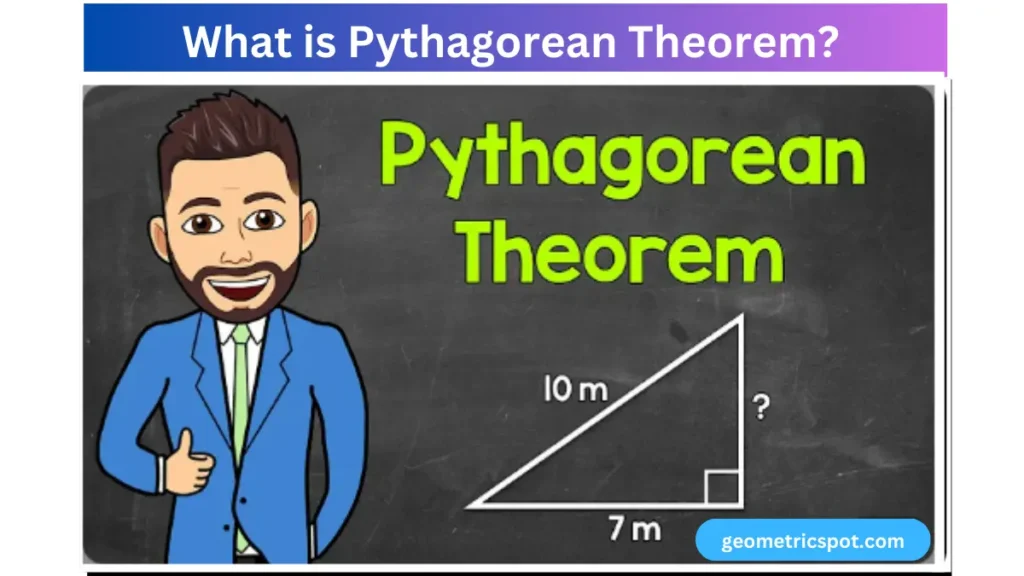
According to the definition: “Pythagorean theorem states that in a right-angled triangle, the square of the length of the hypotenuse is equal to the sum of the squares of the two other sides.
Mathematically, it can be written as:
a^2 + b^2 = c^2
Where,
- a and b are the lengths of the legs which are also known as shorter sides.
- c is the length of the hypotenuse or the bigger side of the right-angled triangle.
How to use Pythagorean Theorem to find the missing sides?
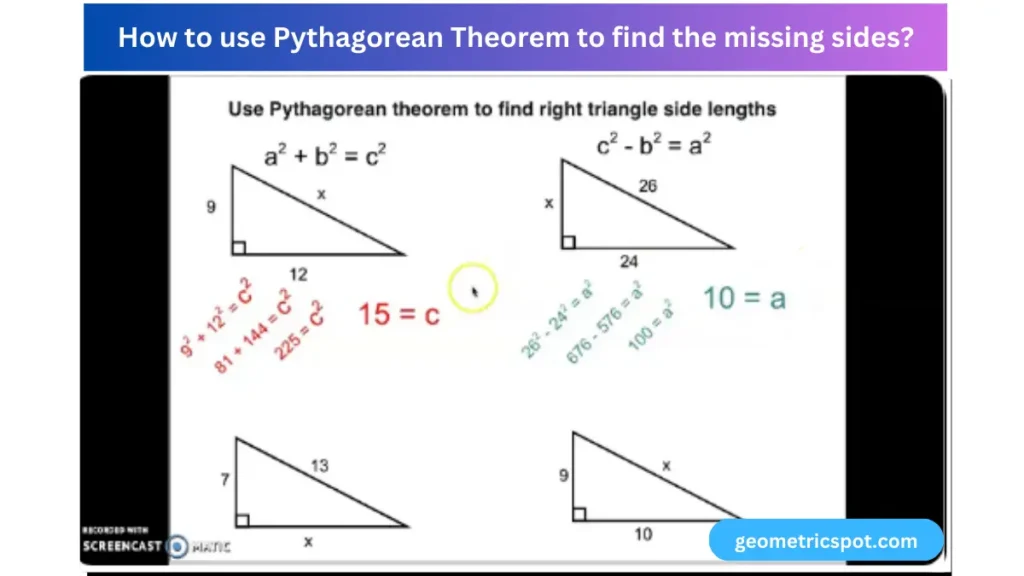
I’ve designed a step-by-step approach and process to use this theorem. You will learn about each and every step to find the missing side lengths by Pythagorean Theorem.
Step#1
Identify the right-angled triangle
First, check and verify that your triangle is a right-angled triangle. In simple words, the angle should be 90°. We can only apply Pythagorean theorem to right angle triangles.
Step#2
Mark and label the sides
Identify the shorter and longer sides and mark them as a,b, and c. We usually represent hypotenuse with c. Hypotenuse is the opposite side of the right angle triangle. Moreover, you can label a and b according to your preferred choice.
Step#3
Apply the Pythagorean theorem
The formula is a^2 + b^2 = c^2. Use this equation to find the missing side. You are free to rearrange them according to the missing side.
Step#4
Solve for the Missing side
How to do a Pythagorean theorem when c is missing? For instance;
If the hypotenuse is missing, use
c = √a^2 + b^2
How to do a Pythagorean theorem when the a and b side is missing? For instance;
If the b side is missing, use
b = √c^2 – a^2
If the a side is missing, use
a = √c^2 – b^2
Step#5
Double check your answer
Once you will find the missing side, double check your calculations and verify the answer by putting values in this equation.
Practical Examples of Pythagorean Theorem to find a missing side
Example : 1 – Finding the hypotenuse
Given a right-angled triangle with both sides is a = 3, b = 4, find the c?
- Identify the sides: a = 3, b = 4, and c is the missing side.
- Apply the equation which is a^2 + b^2 = c^2.
- Put the known values of shorter sides into the theorem. 3^2 + 4^2 = c^2
- Perform the calculation. 9 + 16 = c^2.
- Simplify: 25 = c^2
- The last step is to find the square root. √25 = √c^2.
- Simplify: 5 = c or c = 5.
Example : 2 Finding a leg
Given value of hypotenuse c = 10 in a right-angled triangle. The value for the one shorter leg b = 6. Find the missing leg a?
- Identify the right-angled triangle sides. b = 6, c = 10 and a is the missing side.
- Apply the theorem which is a^2 + b^2 = c^2.
- Rearrange the formula as a^2 = c^ – b^2.
- Put the known values into the theorem. a^2 = 10^2 – 6^2.
- Calculate: a^2 = 100 – 36
- Simplify: a^2 = 64
- Find the square root of: √a^2 = √64
- Final answer is: a = 8
Tips for Mastering the Pythagorean Theorem
- Consistency is the key towards success. Try to solve multiple problems and worksheets on different figures.
- Draw different right-angled triangles and label sides. Use graph paper or digital applications to practice these geometric shapes.
- Put difficult and different values in the Pythagorean theorem. Rearrange the formula and substitute the values.
Visit How Do Geometry Transformations Work | Types of Transformations for more learning.
Why is Pythagorean Theorem important?
Nowadays, it is used by architects, engineers, applied physics, and computer graphics. You can calculate distance, design a structure and map, and solve a real-world problem using right-angled triangles.
Final Words
The Pythagorean theorem is a weapon of geometry used to find the missing sides of a right-angled triangle. Try to use the step-by-step approach which is mentioned above. No matter if you are a teacher, student or a mathematics learner. Learning about Pythagorean theorems helps to explore the real-world of mathematics.
Frequently asked questions
How do we find the missing angle using side lengths?
You need to know about the trigonometric formulas. Here, we will use cosines.
Can we use Pythagorean theorems on all types of triangles?
No, this theorem is only applied to right-angled triangles. It gives us a specific relationship between the three sides of the triangle. One is longer and the other two are shorter.
How to prove the Pythagorean theorem?
Draw a perpendicular from the right-angle vertex to the hypotenuse. So, AB + CD = AC. Substitute the values.