Exploring the Geometry Of Circles: Tangents, Secants & Chords With Examples
Circles are fascinating and unique geometric shapes with mind-blowing and unique properties. They are so dense that mathematicians spent centuries to learn and figure them out.
In this article, we will explore every important aspect of a circle and their properties. Exploring the Geometry Of Circles: Tangents, Secants & Chords With Examples are some of the properties of circles. I’ll break down these unique concepts with practical examples.
What is a circle?
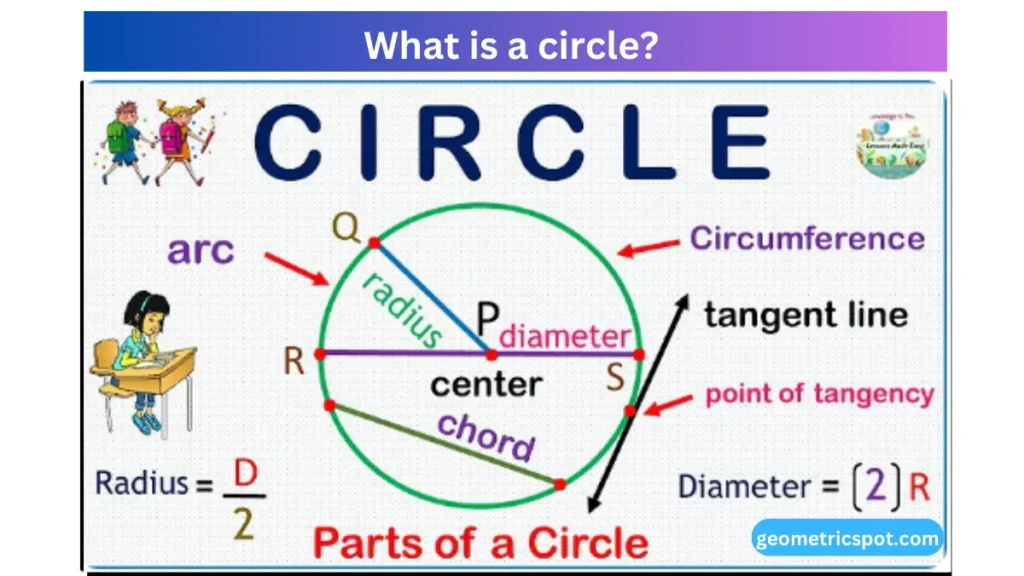
Circle is a two-dimensional shape where all points are located at the same distance from the central point. We called this equal distance from the center of the circle as the radius. On the other hand, the diameter is twice the radius. It starts from one point of the circle and touches the second point. Also try: Izzi Casino.
Basic terms in a circle
Before moving towards chords, tangents and secants. We will learn about some basic concepts of circles. Here are some key terms of circles:
Circle is a two-dimensional shape where all points are located at the same distance from the central point. We called this equal distance from the center of the circle as the radius. On the other hand, the diameter is twice the radius. It starts from one point of the circle and touches the second point. Also download Bet777 and Orion Star 777.
- Radius (r) – The distance from the center of a circle to any point on the circumference.
- Diameter (d) – Diameter is the double of radius. Basically, it’s a line segment passing through the center of the circle and touching two points.
d = 2r
- Circumference (c) – Circumference is the round line around the circle. In simple words, the perimeter of the circle, measured as C = 2πr.
- Arc – A portion or part of the circumference is called arc.
- Sector – A particular region bounded by two radii and an arc between them.
What is a Tangent?
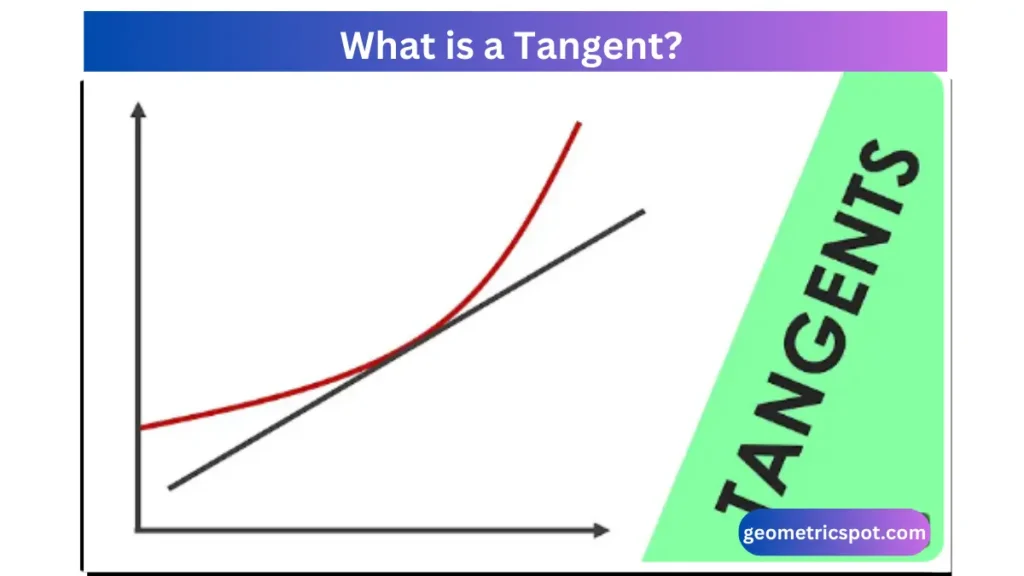
By definition, “A straight line that touches the circle at one point.” It’s called the point of tangency. One of the key properties of tangents is that: they are perpendicular to the radius at the point of tangency.
Key Properties of Tangents
- A tangent is an exact perpendicular to the radius at the point of tangency.
- Whenever you draw a tangent from an external point to a circle, it is equal in length.
Practical Examples of Tangents
Example : 1 Find the length of the tangents
The circle has radius = 5 units, and a point p located 13 units from the center of the circle. Find the length of the tangent from point P to the circle?
- Identify the right triangle by radius, the tangent, and the line segment from the center to the point P.
- Apply the Pythagorean theorem:
r^2 + tangent^2 = distance from center to P^2
- Substitute the values as 5^2 + tangent^2 = 13^2
- Calculate the values: 25 + tangent^2 = 169
- Solve to find tangent: tangent^2 = 144
- Find the root of the tangent.
- Final answer will be tangent = 12 units. So, the missing length of tangent is 12 units.
What is a Chord?
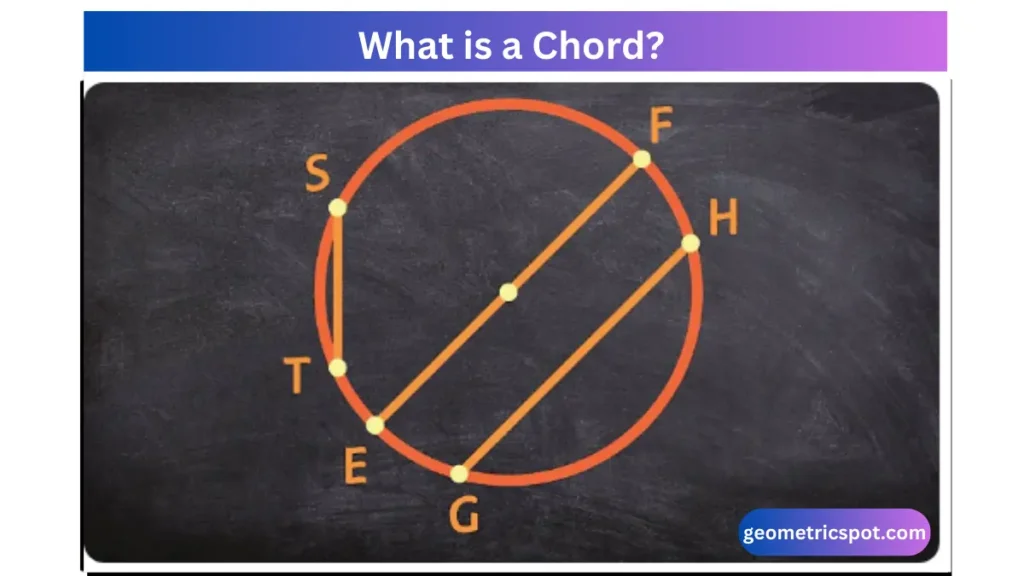
By definition, “A chord is a line segment whose endpoints lie on a circle. Diameter is the longest chord. It passes through the center.
Basic properties of a Chord
- Generally, chords from the center of a circle are equal in length.
- The perpendicular bisector of the chord passes through the center.
- The length of the chord is calculated by a formula.
L = 2√r^2 – d^2
Practical examples of Chord
Example :1 Find the length of a Chord?
Given a circle with radius r =10 units, and a chord 6 units away from the center. Find the length of the chord?
- The chord length formula is:
L = 2√r^2 – d^2
- Substitute the values L = 2√10^2 – 6^2
- Calculate L = 2√100 – 36
- Simplify L = 2√64
- The square root of 64 = 8. Hence, 8*2 = 16.
What is a Secant?
By definition, “A secant is a straight line that intersects a circle at two points. It’s not like a chord. A secant extends beyond the circle.
Basic properties of a secant
- When two secants intersect outside a circle, the product of the lengths of the one segment is equal to the product of the lengths of the other segment.
- One of the interesting theorems is the Secant-tangent theorem. When a secant and a tangent intersect outside the circle. Then, the product of the lengths of the secant segment is equal to the square of the tangent segment.
Practical Examples of Secant
Example : 3 Use the intersecting secant theorem
The given two secants when intersecting outside a circle at a point P, with segment PA = 4 units0, PB = 6 units, PC = 3 units, and find PD?
- Apply the theorem: PA * PB = PC * PD
- Substitute the values: 4*6 = 3* PD
- Calculate 24 = 3*PD
- Simplify: 24/3 = 8
Final Words
See the beauty and complexity of tangents, chords and secants. Mathematics is a vast field but it has interesting properties and fundamental theorems to solve a wide range of geometric and algebraic problems. Whether you are a learner, student or a teacher, you can unwrap the concepts of mathematics to explore the world. It’s not just about solving problems, it’s all about discovering patterns to understand the shape of the world.
Frequently asked questions
What is the circle theorem for tangents and chords?
It states that a tangent is perpendicular to the radius at the point of tangency. All tangents from a common external point are equal. On the other hand, chords are perpendicular from the center and bisects the chords.
Is there any formula for tangents and secants in a circle?
Yes, there are important formulas.
- Tangents-Secants theorem: PA^2 = PB* PC
- Secant-Secant theorem: PA* PB = PC* P