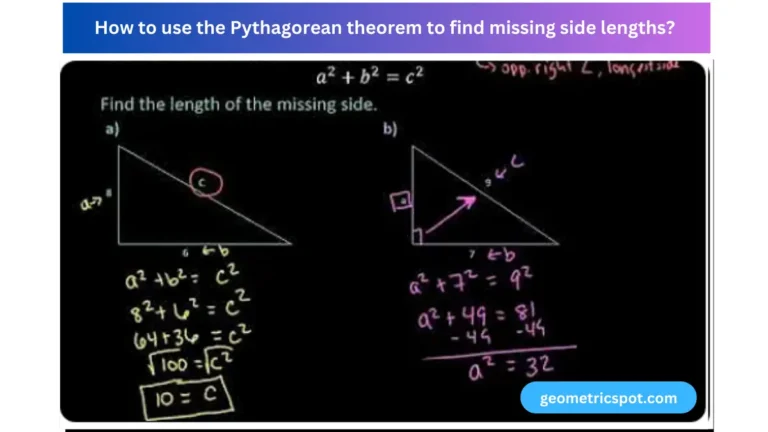
What Actually is Sine, Cosine, and Tangent? Basic, Visualizing & Applications

Understanding sine, cosine, and tangent is fundamental to grasping trigonometry, a branch of mathematics dealing with the relationships between the angles and sides of triangles.

These functions are pivotal in various fields, including engineering, physics, computer science, and even art. Let’s dive into the essence of these What Actually is Sine, Cosine, and Tangent? Basic, Visualizing & Applications and how they are applied.

The Basics of Trigonometry

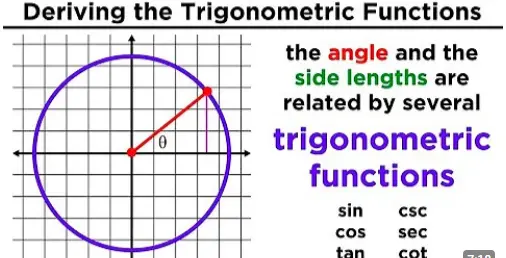
Trigonometry primarily revolves around right-angled triangles, where one of the angles is 90 degrees. The sides of these triangles have specific names:

• Hypotenuse: The longest side opposite the right angle.
• Opposite: The side opposite the angle of interest.
• Adjacent: The side next to the angle of interest and the right angle.

Introducing Sine, Cosine, and Tangent

These trigonometric functions are ratios derived from the lengths of these sides. They help us understand the relationships between the angles and sides of a triangle.

1. Sine (sin)

The sine of an angle is the ratio of the length of the opposite side to the hypotenuse. sin(θ)=OppositeHypotenuse\text{sin}(\theta) = \frac{\text{Opposite}}{\text{Hypotenuse}}sin(θ)=HypotenuseOpposite​

2. Cosine (cos)

The cosine of an angle is the ratio of the length of the adjacent side to the hypotenuse. cos(θ)=AdjacentHypotenuse\text{cos}(\theta) = \frac{\text{Adjacent}}{\text{Hypotenuse}}cos(θ)=HypotenuseAdjacent​

3. Tangent (tan)

The tangent of an angle is the ratio of the length of the opposite side to the adjacent side. tan(θ)=OppositeAdjacent\text{tan}(\theta) = \frac{\text{Opposite}}{\text{Adjacent}}tan(θ)=AdjacentOpposite​

Visualizing Sine, Cosine, and Tangent

To visualize these concepts, consider the following diagram of a right-angled triangle:

In this triangle:

• Hypotenuse (H) is the longest side.
• Opposite (O) is the side opposite to the angle θ\thetaθ.
• Adjacent (A) is the side next to the angle θ\thetaθ.

Let’s label the sides:

• Hypotenuse (H)
• Opposite (O)
• Adjacent (A)

For angle θ\thetaθ:

• sin(θ)=OH\text{sin}(\theta) = \frac{O}{H}sin(θ)=HO​
• cos(θ)=AH\text{cos}(\theta) = \frac{A}{H}cos(θ)=HA​
• tan(θ)=OA\text{tan}(\theta) = \frac{O}{A}tan(θ)=AO​

Applications of Sine, Cosine, and Tangent

These trigonometric functions are not just abstract mathematical concepts; they have practical applications in various fields:

1. Physics: Trigonometric functions are used to describe wave patterns, oscillations, and other phenomena.
2. Engineering: They are essential in understanding forces, motion, and structural design.
3. Computer Graphics: Trigonometry helps in rendering 3D objects and animations.
4. Navigation: Used in GPS technology and to determine distances and directions.
5. Music: Describing sound waves and harmonics.

Understanding Through the Unit Circle

Another powerful way to understand these functions is through the unit circle, a circle with a radius of 1 centered at the origin of a coordinate system. The unit circle allows us to extend the definitions of sine, cosine, and tangent beyond right-angled triangles.

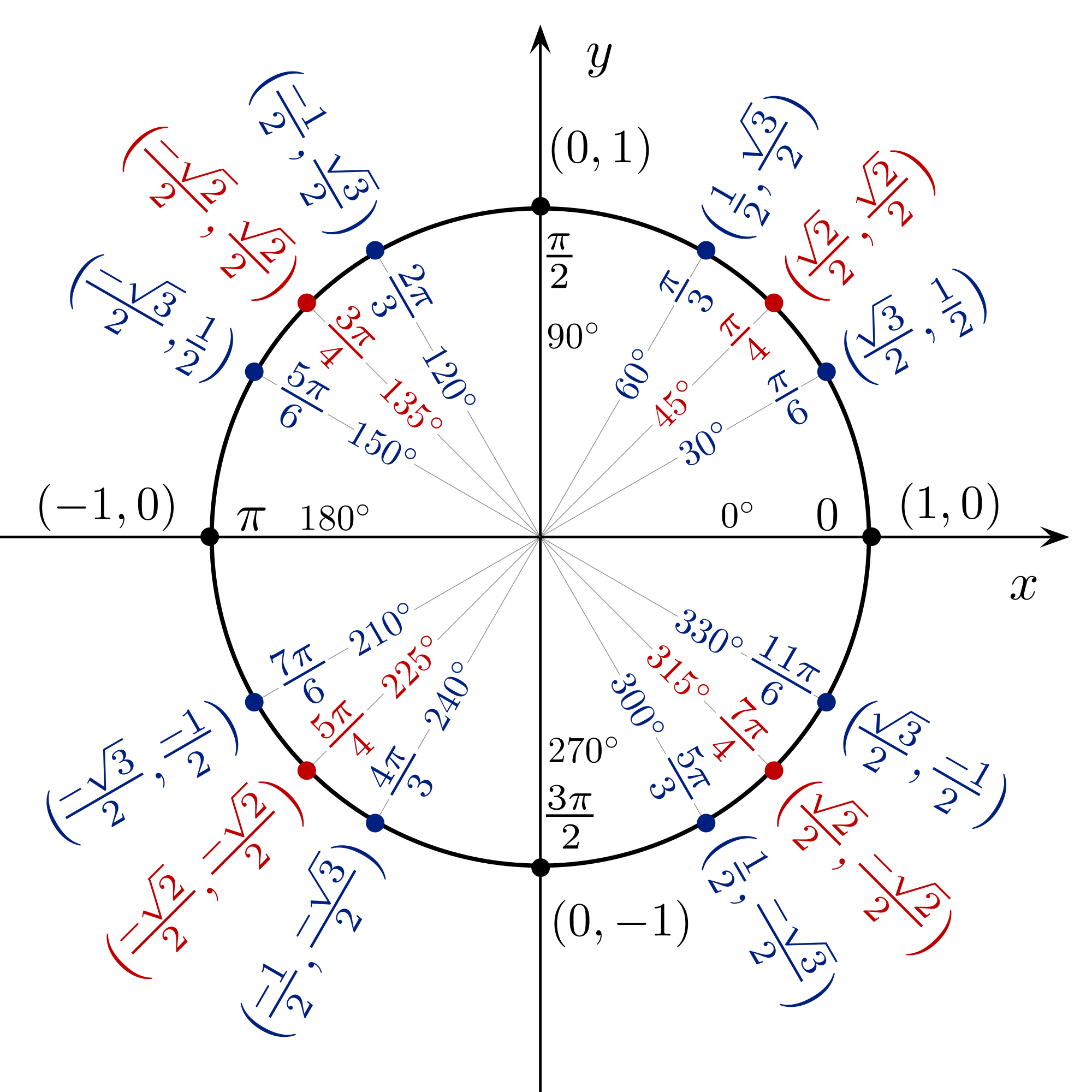
In the unit circle:

• The x-coordinate of a point on the circle is the cosine of the angle.
• The y-coordinate of a point on the circle is the sine of the angle.
• The tangent of the angle is the y-coordinate divided by the x-coordinate (if the x-coordinate is not zero).

Real-World Example: Ferris Wheel

Consider a Ferris wheel as a practical example:

• The height of a passenger at any point can be modeled using sine and cosine functions.
• If the Ferris wheel has a radius rrr and makes a full rotation in TTT seconds, the height hhh of a passenger at time ttt can be expressed as: h(t)=r⋅sin⁡(2πtT)h(t) = r \cdot \sin\left(\frac{2\pi t}{T}\right)h(t)=r⋅sin(T2πt​) This equation shows how the height changes over time, capturing the periodic nature of the wheel’s motion.

Conclusion

Sine, cosine, and tangent are foundational elements of trigonometry with extensive applications across multiple fields. Understanding these functions provides valuable insights into the relationships between angles and sides of triangles, as well as periodic phenomena in the real world. With a solid grasp of these concepts, you can unlock a deeper understanding of both theoretical and applied mathematics.

By mastering the basics and exploring their applications, you can appreciate the profound impact of trigonometric functions in our daily lives and various scientific endeavors.